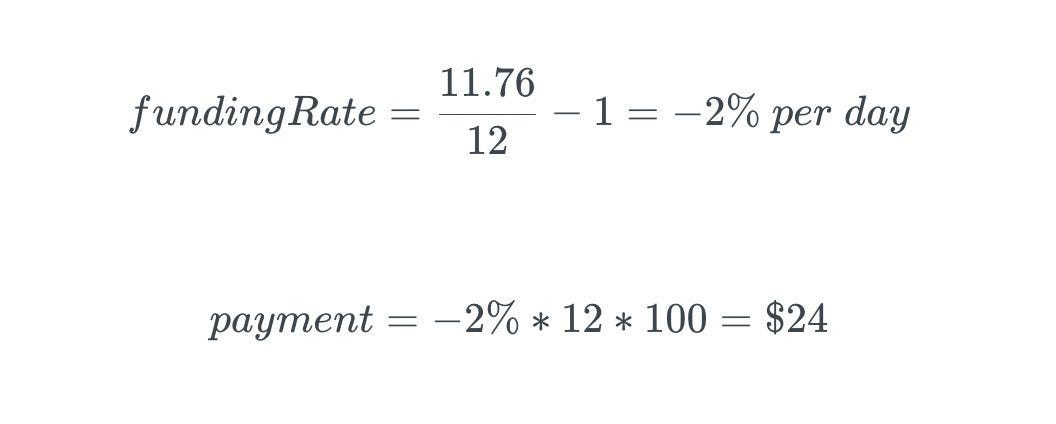Contents
Dated Futures Convergence Mechanism
Contango
Backwardation
Backwardation Case Study — the Merge
Perp Funding Rates
Perp Funding Payments
Funding Payment Example
Conclusion
Synopsis
Dated futures' prices converge with spot prices as expiry nears, offering settlement options like rolling, closing, or holding; cash-settled futures involve credits or debits between parties.
The futures basis represents the price difference between futures and spot prices, while the term structure shows contract pricing across maturities.
Traders examine the slope of the futures curve to identify trading opportunities
Perpetual swaps lack expiry dates and use a funding rate mechanism to maintain price convergence, encouraging traders to adjust positions.
Concentrated liquidity in perpetual swaps leads to tighter spreads, enhanced price discovery, and streamlined trading compared to fragmented dated futures markets.
Dated Futures Convergence Mechanism
In dated futures markets, contract prices converge with spot prices as expiries draw closer. Before maturity, futures positions can be rolled, closed out (via offsetting), or held until settlement. Cash-settled futures are settled via a credit or debit between counterparties on the predetermined expiration date. The futures basis describes the discrepancy between futures contract prices and spot prices.
The futures term structure refers to the pricing of futures contracts across maturities. The slope of the term structure is influenced by factors such as supply, demand, and liquidity. Traders keep tabs on the futures curve to spot trading opportunities; the futures curve is a strong indicator of sentiment around commodities (and commodity monies).
Contango
Contango is the default state of a futures curve and indicates futures trading at a premium relative to spot prices. Contango occurs when the futures price of an asset is higher than its current spot price. This typically happens when the market anticipates an increase in the asset's value over time.
In a contango market, traders holding long positions in futures contracts may choose to sell their contracts and buy the underlying asset at a lower spot price, making a profit. This selling pressure drives the futures price down, bringing it closer to the spot price.
Backwardation
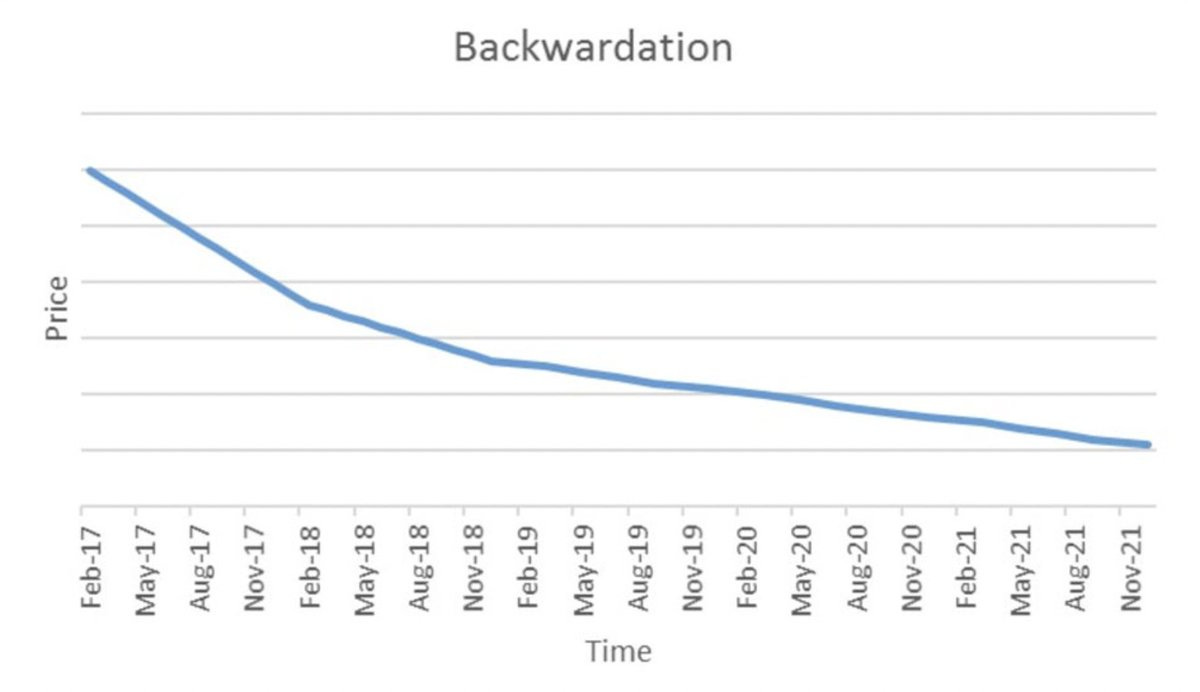
The less common, backwardated (inverted) futures curve is indicative of futures trading at a discount relative to spot prices. Backwardation occurs when the futures price is lower than the spot price, indicating a potential decrease in the asset's value.
In a backwardation market, traders with short positions may choose to buy back their contracts and sell the underlying asset at a higher spot price, again driving the futures price closer to the spot price.
Backwardation Case Study — the Merge
Looking back at the pre-merge $ETH futures term structure, we can see that the curve was in backwardation, indicating a preference for spot $ETH over $ETH futures — likely due to $ETH PoW airdrop eligibility being conferred by holding spot but not futures.
Perp Funding Rates
Now, onto perpetual contracts and their novel convergence mechanism. Unlike dated futures, perps do not expire and have no settlement or delivery date, so an alternative mechanism must exist to incentivize the tracking of the underlying asset.
Perpetual swaps are similar to futures contracts, but they do not have an expiration date. To maintain price convergence with the underlying asset, perpetual swaps use a mechanism called the "funding rate." The funding rate is an interest rate that long or short-position holders pay or receive periodically. It incentivizes traders to close or open positions, thus ensuring the perpetual swap price stays close to the spot price.
Funding rates are the tether that keeps the price of perpetual contracts in line with spot prices. These rates are expressed as positive or negative percentages, contingent upon the magnitude of divergence between the price of the perp relative to the underlying asset.
When the funding rate is positive, the perp trades at a higher price than its underlying asset. Conversely, a negative funding rate is indicative of the perp trading lower than its underlying asset.
Funding Payments
Funding payments are determined by the funding rate. Longs pay shorts when the funding rate is positive, and shorts pay longs when the funding rate is negative. The result is a rubber-band effect that enforces a degree of parity between the price of a perp and spot price.
The funding rate is determined by the difference between the perpetual swap price and the spot price. When the perpetual swap price is higher than the spot price (positive funding rate), long-position holders pay a funding fee to short-position holders. This incentivizes traders to close long positions or open short positions, driving the perpetual swap price down toward the spot price.
Zeta does not pay or collect funding payments; rather they are exchanged directly between traders — pro rata to the size of their positions (think of the exchange as Switzerland when it comes to funding payments).
Funding payments on Zeta are extrapolated from a 24hr realization period. That is, the expected payment traders could expect to be credited or debited on a daily basis.
Example
Chad goes long 100 SOL-PERP contracts and keeps his position open for 24hrs. Over that time, the impact midpoint is $11.76, and the SOL oracle price is $12. The resulting funding rate would be -2% and Chad would receive $24/day. Nicely done, Chad.
Conclusion
In a dated futures contract, the buyer agrees to purchase an asset at a specified price on a predetermined future date. As the expiration date approaches, the futures price converges to the spot price of the underlying asset.
Perpetual swaps are similar to futures contracts, but they do not have an expiration date. To maintain price convergence with the underlying asset, perpetual swaps use a mechanism called the "funding rate." The funding rate is an interest rate that long or short-position holders pay or receive periodically. It incentivizes traders to close or open positions, thus ensuring the perpetual swap price stays close to the spot price.
Dated futures contracts and perpetual swaps use different mechanisms to ensure price convergence with the underlying asset. Dated futures rely on the natural convergence of futures prices as the contract expiration date approaches. Perpetual swaps, on the other hand, use funding rates to incentivize traders to adjust their positions, thus maintaining the perpetual swap price close to the spot price of the underlying asset.